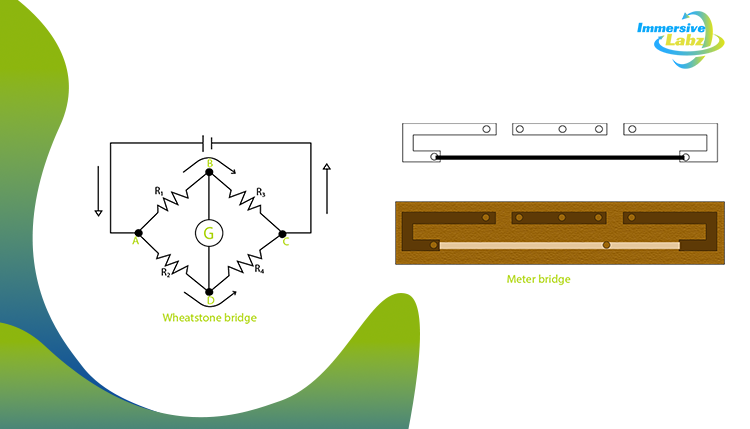
The meter bridge, also known as the slide wire bridge, consists of a 1-meter-long wire of uniform cross-sectional area fixed on a wooden block. A scale is attached to the block. Two gaps are formed on it by using thick metal strips in order to make the Wheatstone Bridge.

The meter bridge operates using the Wheatstone principle. Here, four resistors P, Q, R, and S are connected to form the network ABCD. Terminals A and C are connected to a battery, and terminals B and D are connected to a galvanometer.

In the balancing condition, there is no deflection on the galvanometer. Then, $$\frac{P}{Q}=\frac{R}{S}$$

If a resistance wire of unknown resistance 𝑋 is introduced in the right gap of the meter bridge and the high resistance 𝑅 is introduced in the left gap of the meter bridge, then as the jockey slides over the bridge wire, it shows zero deflection at the balancing point (null point).

If the balancing length is 𝑙, then according to the Wheatstone principle, we have $$\frac{X}{R}=\frac{l}{100-l}$$

The unknown resistance is given by $$X=R \frac{l}{100-l}$$

Using the above formula, the unknown resistance of two wires is calculated. Then, these wires are connected in the gap of the meter bridge as their series and parallel combinations.

Law of Series Combination

A circuit is said to be connected in series when the same amount of current flows through the resistors. The voltage across each resistor is different, and the current across each resistor is the same.

In the above diagram, the series equivalent is given by $$R_s=R_1+R_2+\cdots+R_n$$

Law of Parallel Combination

A circuit is said to be connected in parallel when the voltage is the same across each resistor. In such circuits, the current is branched and recombined when the branches meet at a common point.

In the above diagram, the parallel equivalent is given by $$\frac{1}{R_p}=\frac{1}{R_1}+\frac{1}{R_2}+\cdots+\frac{1}{R_n}$$

Using these laws, the equivalent resistance of series and parallel combinations of resistances is calculated and verified.

Requirements for this Science Experiment

⦁ Meter Bridge ⦁ Jockey ⦁ Resistance Box ⦁ Plug Key ⦁ Battery ⦁ Resistance Wire